Question
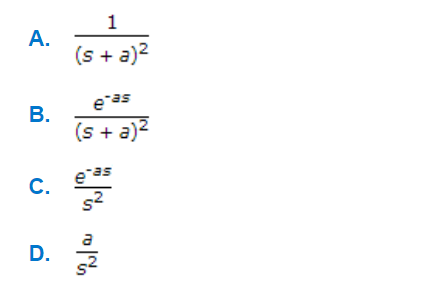
a.
A
b.
B
c.
C
d.
D
Posted under Electronics and Communication Engineering
Interact with the Community - Share Your Thoughts
Uncertain About the Answer? Seek Clarification Here.
Understand the Explanation? Include it Here.
Q. The Laplace transform of unit ramp function starting at t = a is
Similar Questions
Explore Relevant Multiple Choice Questions (MCQs)
Q. If i(t) is a time varying current, then following is
View solution
Q. For the discrete time system of the given figure
View solution
Q. The following signal is
View solution
Q. If H(z) is given by following equation, the poles of H(z) are at
View solution
Q. The function x(t) is shown in the given figure. Even and odd parts of a unit step function ∪ (t) are respectively,
View solution
Q. Fourier transform of -jt f(t) is
View solution
Q. DTFT (Discrete time Fourier transform) of x[n] = aⁿ∪[n] for -1 < a < + 1.
View solution
Q. The given figure shows a discrete time system consisting of a unit delay system, a multiplier and a summer, such that y(k) = x(k - 1) + 0.5 x(k). This system
View solution
Q. The joint probability function of two discrete random variable X and Y is given below.
Then E(y) is
View solution
Q. The joint probability function of two discrete random variable X and Y is given below.
Variance σ² will be
[Hint: σ² = E(X²) - μ² ⇒ E(X²) - (E(X))²]
View solution
Q. A probability density function is given by p(x) = Ke^(-x²/2) for -∞ < x < ∞ , The value of K should be
View solution
Q. The Laplace transform of f(t) is
View solution
Q. In the given figure 15.6 shows a series, R - C circuit fed by a current source i(t). There is an initial voltage v₀ across the capacitor. The system
View solution
Q. If Laplace transform of f(t) is F(s), then
View solution
Q. Which of the following is the correct Laplace transform of the signal shown in the given figure
View solution
Q. If v(t) is a time varying voltage, then following is
View solution
Q. The data of speed of train V and resistance to motion R is given below. The law R = a + bV² is of the form
View solution
Q. If H(z) is given by following equation, then system is
View solution
Q. If F(s) = 10 / s²+4s+4, f(t) =
View solution
Q. Consider z transform of a signal as given below, then the response will be
View solution
Recommended Subjects
Are you eager to expand your knowledge beyond Electronics and Communication Engineering? We've handpicked a range of related categories that you might find intriguing.
Click on the categories below to discover a wealth of MCQs and enrich your understanding of various subjects. Happy exploring!