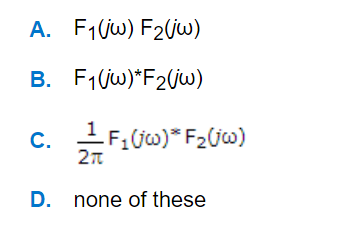
Q. A wave f(t) has half wave symmetry and time period equal to T. Then

View solution

Q. The two inputs to an analogue multiplier are x(t) and y(t) with fourier transforms X(f) and Y(f) respectively. The output Z(t) will have a transform Z(f) given by

View solution

Q. If v(t) = 0 for t < 0 and v(t) = e^(-at) for t ≥ 0, V(jω) =

View solution

Q. Consider the following equation.

View solution

Q. Which of the following can be impulse response of a casual system?

View solution

Q. The Laplace transform of sin ∝ t is

View solution

Q. Fourier transform of the unit step function (i.e., u(t) = 1 for t ≥ 0 and u(t) = 0 for t < 0) is

View solution

Q. The dirac delta function δ(t) is defined as

View solution

Q. Laplace transforn of sin (ωt + a) is

View solution

Q. If x^k = 2^k for k ≤ 0 and xk = 0 for k ≥ 0, Z transform of the sequence x is

View solution

Q. Final value theroem is for sequence x[n] is

View solution

Q. The Laplace transform of (tⁿ-1) where n is integer is

View solution

Q. The data about p the pull required to lift a weight wby a pulley block is given below. The linear law p = a + bw is

View solution

Q. Average power for following signal is

View solution

Q. Which is the Laplace transform of x(t) = -e^(2t) ∪(t) ⊕ t ∪(t)?

View solution

Q. For a signal x(t) the F.T. is X(f). Then inverse F.T of X(3f + 2) is given by

View solution

Q. An experiment is repeated N times. One event A occurs Nᴀ times. Then

View solution

Q. If f(t) is an even function, F(jω) =

View solution

Q. If Laplace transform of f(t) is F(s), then £[tf(t)] =

View solution

Q. The minimum sampling frequency in sample/sec. required to reconstruct the following signal from its samples without distortion would be

View solution